Argand Plane
As we know that (x,y) are a pair of numbers which can be depicted on the XY plane, where x is known as the abscissa, whereas, y is known as the ordinate. Likewise, also the representation of the complex numbers can be done on the plane, known as the Complex Plane or the Argand Plane.
Identical to or related to X and Y-axis in 2-D geometry, there exist 2 axes in the Argand Plane, which are:
- The horizontal axis is referred to as the Real axis
- The vertical axis is referred to as the Imaginary axis
“X+iy”, the complex number corresponds or correlates with the ordered pair i.e. (x,y) which is geometrically represented as a unique point (x,y), lying in an XY-plane.
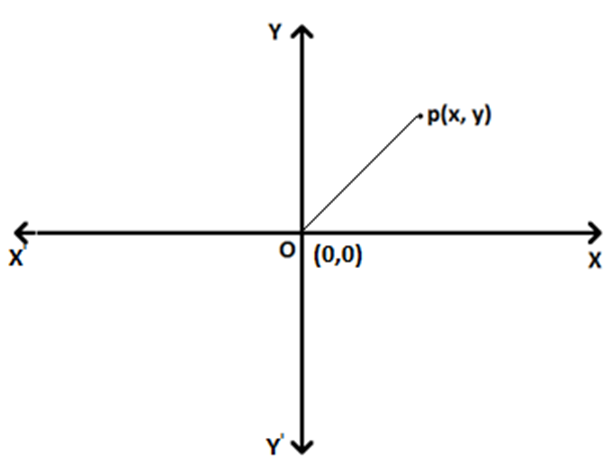
For example,
2+3i, the complex number corresponds to (2,3) which is a geometrically ordered pair.
In a similar way, -3+2i corresponds or correlates with ordered pair —> (-3,2)
- Complex numbers, represented in 0+ai form, where “a” is a real number which lies on the imaginary axis
- Complex numbers, represented in a+0i form, where “a” value is real which lies on the real axis.
It’s pretty obvious that modulus of x+iy complex number |x+iy|, √x²+y² is known as the distance between the point (x,y) and the origin (0,0).
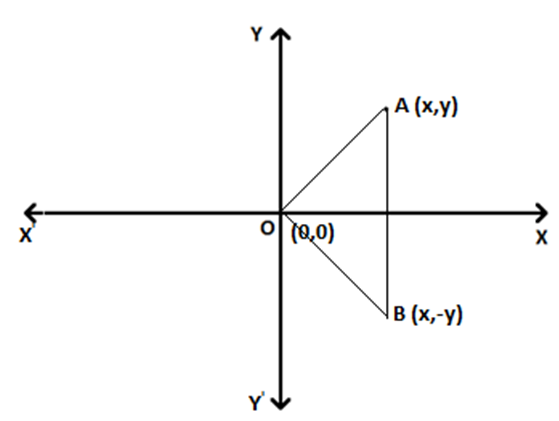
- z = x+iy’s conjugate will be z = x-iy that is expressed as (x,-y) in Argand plane. The point (x,-y) reflects a mirror image of the point (x,y) over the real axis, lying in the Argand plane.
Polar representation of complex numbers:
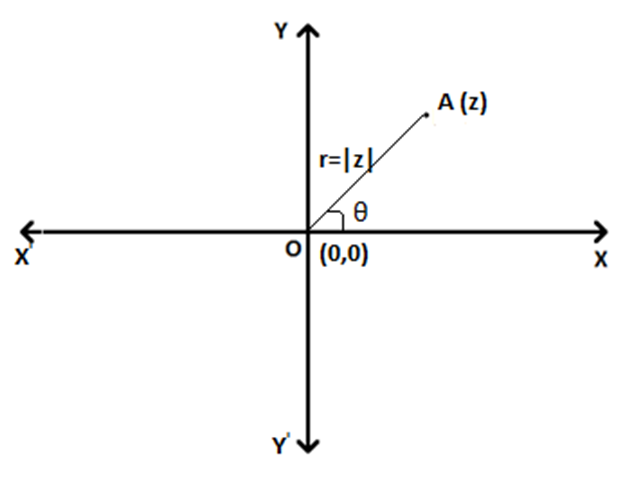
The Polar Representation
We are having various Coordinate system types and one of them is called the – Polar Coordinate system. This Coordinate system is referred to as the “mutually perpendicular lines”. The origin present is known as the “Pole”. And the x-axis is called “Initial Line”.
From any point, measuring the position of that particular point by measuring the line’s length which links it to the origin point plus the angle created by the line with a particular axis.
Let’s say, if φ & r value is known, then, you can locate the position of point P. These are referred to as the polar coordinates, r & φ.

Likewise, if you know a complex number’s Argument lying in Argand Plane & OP length, then, we can find the assumed or the said number.
Let’s take OP = r and also, knowing that |z| = OP = r; where, z = x + iy
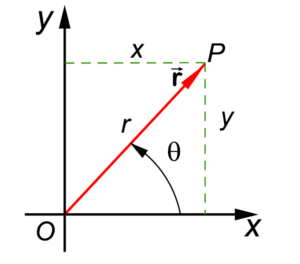
P’s coordinates are (x, y). So, in a right-angled triangle, you can observe that x = r cos(θ) & y = r sin(θ).
Therefore, you can write, z = OP. cos(θ) + OP. sin(θ) = r. cos(θ) + r. sin(θ) = r [cos(θ) + sin(θ)]. And thus, this is the “Polar representation of the complex number”, or,
z = x + iy, is represented as – z = r cosθ + ir sinθ = r(cosθ + i sinθ), that is known as the “Polar Form of Complex Numbers.”z = x + iy which is having:
|z| = r & Arg(z) = θ; In this case, |z| = r = x²+y² is known as the modulus of θ & z, which are known as the amplitude or argument of z, represented as Arg z.
Now, ∵ Tan θ = Sin θ / Cos θ
∴ x = r sin(θ) / r cos(θ) = tan θ
And hence, θ = tan-1(y/x)
So, with the use of this relation, you can find the “Argument of a complex number”.
Also, from the above conclusion, |z| = r & Arg(z) = θ – For any z complex number which is non-zero, there correlates single value of θ, in [0,2π) interval.
Further, in a different interval, having length = 2π; Let’s say, considering -π < θ ≤ π interval then, θ value is known as z’s principal argument.