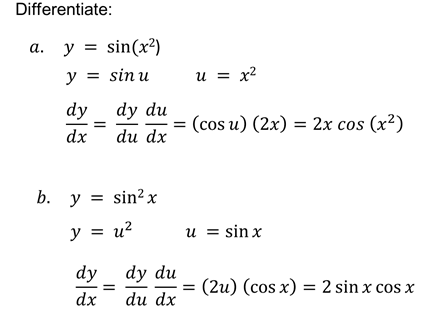A polynomial of degree n has at most n roots such that the cubics have at most 3 roots; quadratics (degree 2) have at most 2 roots and so on. Linear equations area slight exception in that they always have one root. Constant equations have constant integers. The first derivative of a polynomial function of degree n is a polynomial of degree n–1. The roots of the derivative are the critical points of the original polynomial under consideration. The 2nd derivative has degree n–2, and its roots are the potential inflexion points of the original polynomial. Likewise, a polynomial function of degree n has at most n–1 critical points and at most n–2 inflexion points in a graphical point of view.
For studying the derivatives of a polynomial function, we differentiate each term separately. Recall the basic derivative rules to solve the derivative of xn, where n is an integer. These rules are valid for any real number.
Basic Derivates Rules

Consider the example below:
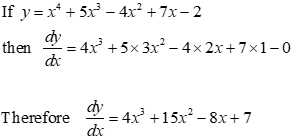
We combine differentiation rules to solve polynomial derivatives.
Ex. Find the derivative of

We combine the power rule, chain rule and quotient rule to solve this derivation:
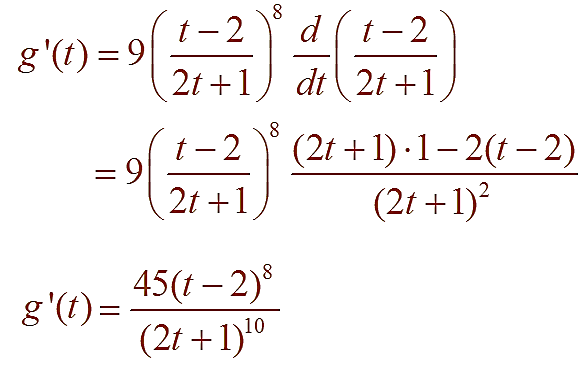
Ex. Differentiate y = (2x + 1)5 (x3 – x + 1)4
In this example, we use the product rule before implementing the chain rule as opposed to the previous example.
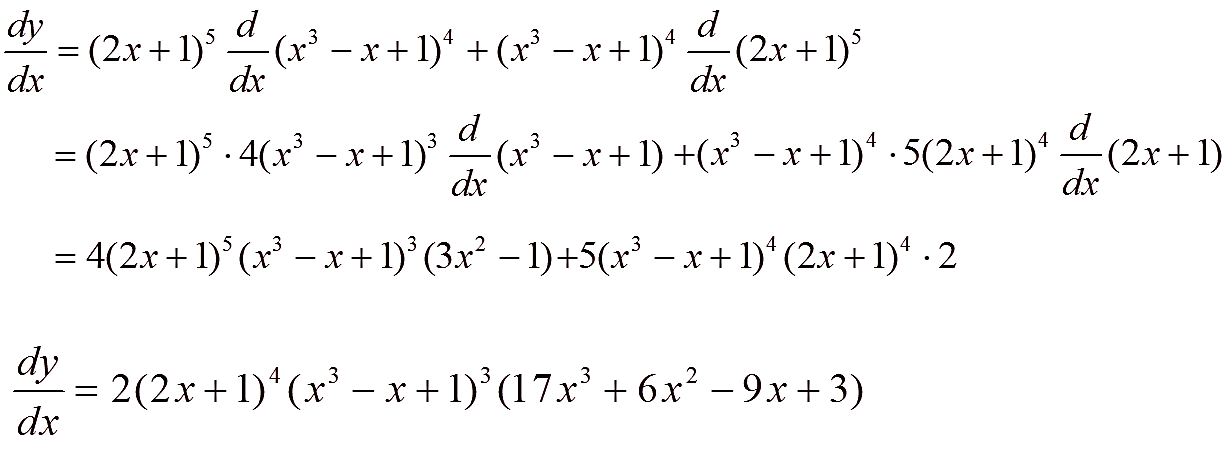
When we talk about the function f defined for all real numbers x by 𝑓(𝑥)=sin𝑥, it is understood that sin𝑥 means the sine of the angle whose radian measure is 𝑥.A similar convention holds for the other trigonometric functions such as cos, tan, csc, sec, and cot. The sketch of the graph of the function f(x) = sin x and using the interpretation of f’(x) as the slope of the tangent to the sine curve in order to sketch the graph of f’ can be seen below:
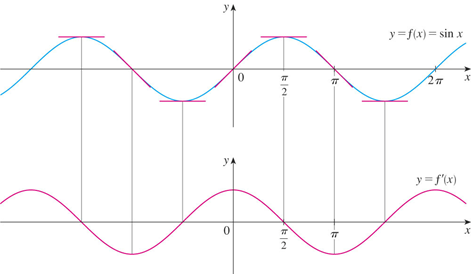
The graph of f’ may look the same as the cosine curve.
From the definition of a derivative, we have:
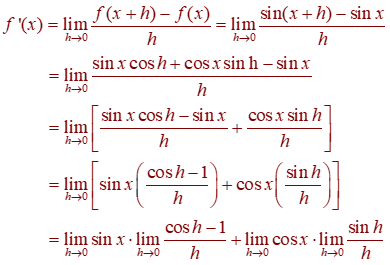
Since we assume x as a constant for computing a limit as h → 0, we obtain:

So, the following rule is valid for the derivative of the sine function:
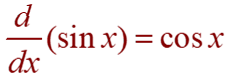
Definition of derivative can also be used to differentiate the tangent function. However, it is easier to use the Quotient Rule together with formulas for derivatives of as follows.
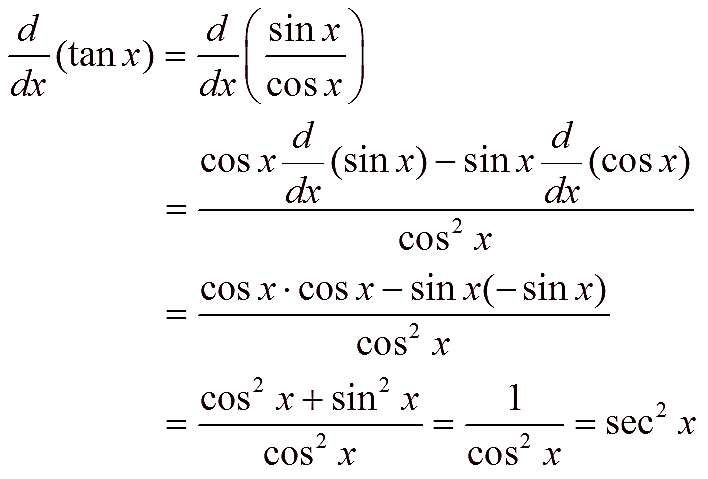
The differentiation formulas for trigonometric functions are tabulated here. Note that, they are valid only when x is measured in radians.
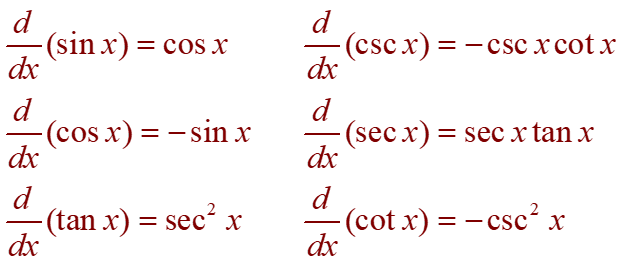
Ex.
Differentiate
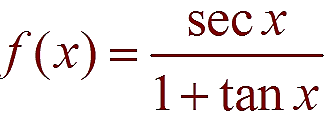
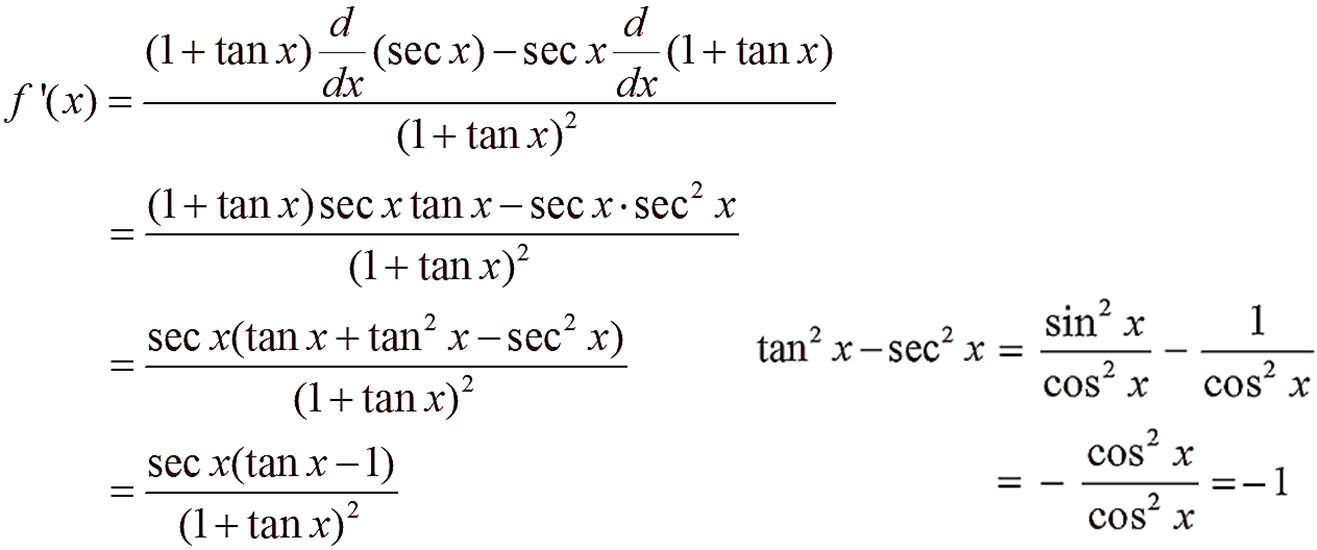
![]()
Because sec x is never 0, f’(x) = 0 when tan x = 1.This occurs at specific points when x = nπ +π/4, where n is an integer.
Ex.