Conic sections: Hyperbola − a point, a straight line and a pair of intersecting lines as a degenerated case of a conic section.
When we pull the two foci of an ellipse so far apart that they moved outside the ellipse, the hyperbola, another conic section is formed. Although ellipses and hyperbolas have completely different shapes, their definitions and equations are comparable. For the hyperbola, instead of using the sum of distances from two fixed foci, as in the case of an ellipse, we use the difference from the two fixed foci.
A hyperbola is defined as a set of points P(x, y) in a plane where the difference of the distances from P to fixed points F1 and F2, the foci, is considered constant: d = |PF1 – PF2 |, where d stands for the constant difference. The transverse axis is the line segment joining the vertices and the midpoint of the transverse axis is the centre of the hyperbola.
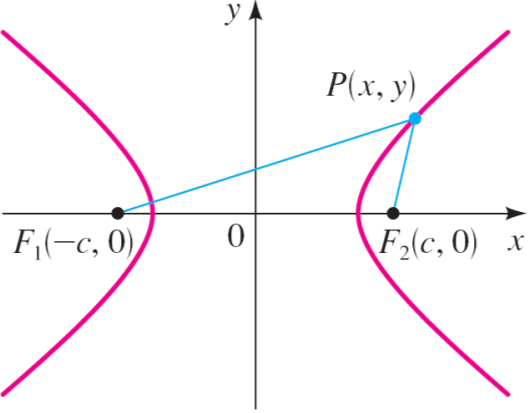
As in the case of the ellipse, we get the simplest equation for the hyperbola by
placing the foci on the x-axis at (±c, 0). By definition, if P(x, y) lies on the hyperbola, then either d(P, F1) – d(P, F2) or d(P, F2) – d(P, F1) is equal to 2a, a positive constant.

Example 1: Find the constant difference for a hyperbola with foci F1 (–8, 0) and F2 (8, 0) and the point on the hyperbola (8, 30).
Recall that the definition of the constant difference of a hyperbola: d = |PF1 – PF2 |
Distance Formula:
![]()
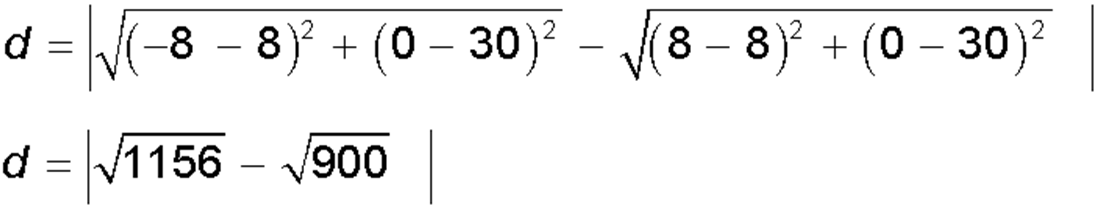
The constant difference and distance are 4.
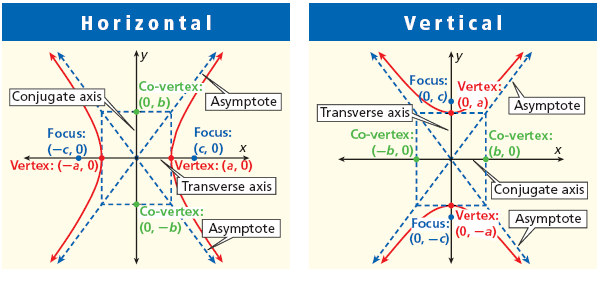
As the graphs above show, a hyperbola contains two symmetrical parts called branches. A hyperbola also has two axes of symmetry. The transverse axis of symmetry shows the vertices and, and when extended, it also contains the foci of the hyperbola. The endpoints of the transverse axis in the hyperbola are its vertices. The conjugate axis of symmetry separates the two branches of the hyperbola in symmetry. It is the line segment of length 2b joining (h, k + b) and (h, k – b) when the transverse axis is horizontal, and the line segment of length 2b joining (h + b, k) and (h – b, k) when the transverse axis is vertical. The co-vertices are noted as the endpoints of the conjugate axis. The transverse axis is not always longer than the conjugate axis and the size depends on the shape of the hyperbola.
Eccentricity is defined by the distance from centre to focus and vertex as follows:
$e=\frac{distance from center to a focus}{distance from center to a vertex}$
$e>1$ iff the conic is a hyperbola because of c > a. It is important to remember that the eccentricity is large, the
branches of the hyperbola are nearly flat. When the eccentricity is close to 1, the branches of the hyperbola are more narrow in shape.