Rigid body:
To understand the rotational motion, first, we will go through the concept of angular position, velocity, acceleration, and centripetal acceleration
The rigid body is a body with a perfectly defined and unchanging shape and the distance between any two particles within the body remains constant.
We will consider rigid bodies having definite shape and size and are capable of having both rotational and translational motion.

Figure: 19.a
Angular velocity
Consider a rigid body rotating about a fixed axis through point O.
Figure: 19.b
While the body is rotating, each and every point in the body moves in a circle with their center lying on the axis of rotation and every point moves through the same angle during a particular interval of time.
Consider the position of a particle say $i^{th}$ particle at point $P$ at a distance $r_{i}$ from point O and it makes an angle $\theta _{i}$ with some reference line say OX.
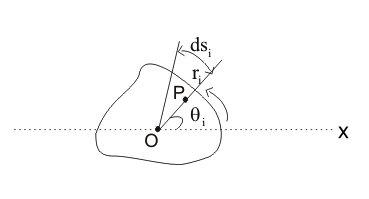
Figure: 19.c
If the particle moves a small distance $ds_{i}$ in time $dt$ then, $ds_{i}=v_{i}dt$
Where $v_{i}$ is the speed of the particle.
$d\theta $ is the angle subtended by an arc of length $ds_{i}$ on the circumference of a circle of radius $r_{i}$, therefore,$d\theta =ds_{i}/r_{i}=v_{i}dt/r_{i}$ ……….. (1)
The distance $ds_{i}$ would vary from particle to particle but angle $d\theta $ swept out in a given time remains the same for all the particles.
So, $d\theta /dt$ is the same for all particles of the rigid body and known as angular velocity $\omega $ of the rigid body.$\omega =d\theta /dt$
So, from equation (1) we get,
$v_{i}=r_{i}d\theta /dt=r_{i}\omega $ …….. (2)
This shows that the velocity of the $i^{th}$ particle of the rigid body is related to its radius and the angular velocity of the rigid body.
The unit of angular velocity is radian per second ($rad s^{-1}$).
Angular acceleration:
Angular acceleration is the rate of change of angular velocity with respect to time.
$\alpha =d\omega /dt=d^{2}\theta /dt^{2}$ ………….. (3)
The unit of angular acceleration is ($rad s^{-2}$) and it is a vector quantity like Angular velocity.
Differentiating equation (2) with respect to t,
$a_{i}=dv_{i}/dt=r_{i}d^{2}\theta /dt^{2}=r_{i}\alpha $
The above equation shows the relationship between the tangential components of the linear acceleration of a point and the angular acceleration of a point.
We also know each particle in the rigid body has the radial linear acceleration component $v^{2}/r$. Therefore, $a_{c}=v_{i}^{2}/r_{i}=r_{i}\omega ^{2}$
This acceleration $a_{c}$ pointing inwards towards the radial line and known as centripetal acceleration.
So, total acceleration can be expressed as, $a=\sqrt{a_{c}^{2}+a_{i}^{2}}$.
Rotation with constant angular acceleration:
When a rigid body rotates with constant acceleration we have,
$\alpha =d\omega /dt=$ constant
or, $d\omega =\alpha dt$
Integrating the above equation, we get,
$\omega =\alpha t+c$
At t = 0, $\omega =\omega _{0}$ then $c=\omega _{0}$
Then, $\omega =\alpha t+\omega _{0}$ ……………………(4)
We have angular velocity,
$\omega =d\theta /dt$
Or, $d\theta =\omega dt$
Or, $d\theta =(\alpha t+\omega _{0}) dt$
Integrating the above equation, we get
$\theta =\omega _{0}t+1/2 \alpha t^{2}+c_{1}$
At t = 0, $\theta =\theta _{0} then c=\theta _{0}$
Then,
$\theta =\omega _{0}t+1/2 \alpha t^{2}+\theta _{0}$ ……………………… (5)
Now, $\alpha $can be written as,
$\alpha =\omega \frac{\alpha }{\omega }=\omega \frac{d\omega /dt}{d\theta /dt}=\omega \frac{d\omega }{d\theta }$
Or, $\alpha d\theta =\omega d\omega $
Integrating, we get,
$\alpha \theta =\omega ^{2}/2+c_{2}$
At t = 0, $\theta =\theta _{0}and \omega =\omega _{0} then c_{2}=\alpha \theta _{0}-\omega _{0}^{2}/2$
Therefore,
$\alpha \theta =\omega ^{2}/2+\alpha \theta _{0}-\omega _{0}^{2}/2$
Or, $\alpha (\theta -\theta _{0})=1/2(\omega ^{2}-$$\omega _{0}^{2}$)
Or, $(\omega ^{2}-$$\omega _{0}^{2})=2\alpha (\theta -\theta _{0})$ ……………………… (6)
Equations (4), (5) and (6) give the equation of motion with constant angular acceleration.