Capacitor is a two-terminal electric component that has the ability or capacity to store energy in the form of electric charge. The ability of a capacitor to store the energy in the form of an electric charge is known as Capacitance.
Symbol of a capacitor:
Figure:9.a
Charging a capacitor: The plates of the capacitor are connected to the terminals of a battery to transfer charge from one conductor to another.
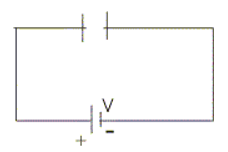
Figure:9.b
Plate connected to the positive terminal of the battery becomes positively charged and the plate connected to the negative terminal of the battery becomes negatively charged. Both plates have equal amount of opposite charge.
Once the capacitor is fully charged, the potential difference between the conductors due to their equal and opposite charges becomes equal to the potential difference between the battery terminals.
For a given capacitor,
Charge $Q$is proportional to the potential difference of $V$.
So, $Q \alpha V$
Or, $Q =CV$
Where $C$ IS the proportionality constant and known as capacitance of the capacitor.
Capacitance of any capacitor depends on the shape, size and geometrical arrangement of the conductors.
The SI unit of capacitance is farads(F).
$1F=$1 coulomb/volt
Parallel plate capacitor:
A parallel plate capacitor consists of two parallel conducting plates separated by a small distance.
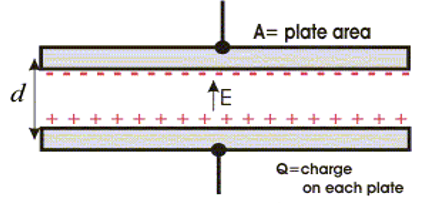
Figure:9.c
Suppose two plates of a capacitor has equal and opposite charge $Q$ on them. If $A$ is the area of each plate, the surface charge density on each plate is $\sigma =Q/A$
Electric field between two oppositely charged plates is $E=\sigma /\varepsilon _{0}=Q/A\epsilon _{0}$
The potential difference between the plates is$V=E.d$
Or, $V=Qd/A\epsilon _{0}$
Where, $d$ is the separation between the plates.
Therefore, the capacitance of a parallel plate capacitor in vacuum is $C=Q/V$
Or, $C=Q\frac{ A\epsilon _{0}}{Qd}=\epsilon _{0}A/d$
The above equation shows that the capacitance is independent of charge on the capacitor but depends on the area of the plates of the capacitor and the distance between the plates.
Cylindrical capacitor:
A cylindrical capacitor is made up of a conducting cylinder or wire of radius $a$ (say) surrounded by another concentric cylindrical shell of radius $b$ (say). Let L be the length of both the cylinders and charge on inner cylinder is and on outer cylinder is $+Q$ and on the outer cylinder is $-Q$.
Imagine a Gaussian surface of radius $r$ and length $L_{1}$
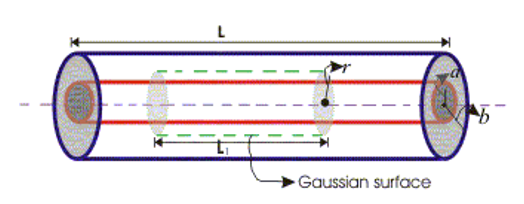
Figure:9.d
Now Electric flux is given by,$\varphi =E . {A}=E. 2\pi rL_{1}=Q/\epsilon _{0}$
Then, $E. 2\pi rL_{1}=Q/\epsilon _{0}$
Therefore, the electric field between the cylinders is
$E=Q/2\pi rL\varepsilon _{0}$, Where $L$ = length of the cylinder
$V=_{a}^{b}{}Q/2\pi rL\varepsilon _{0} dr$
$V=\frac{Q}{2\pi L\varepsilon _{0}}_{a}^{b}{}\frac{dr}{r}$
Or, $V=\frac{Q}{2\pi L\varepsilon _{0}}ln(b/a)$
Thus capacitance of the cylindrical capacitor is given by,
$C=Q/V=\frac{2\pi L\varepsilon _{0}}{ln(b/a)}$
Capacitors in parallel and series:
Parallel combination:
Let us consider two capacitors connected in parallel between two points A and B
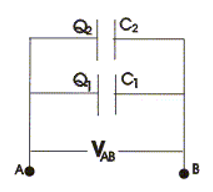
Figure:9.e
If $V_{A}$and $V_{A}$ are the common potential at left side plates and right side plates respectively, the potential difference $V_{AB}=V_{A}-V_{B}$would be the same for both capacitors.
If $Q_{1}$and $Q_{2}$are the charges on both the capacitors, then,
$Q_{1}=C_{1}V_{AB}$and $Q_{2}=C_{2}V_{AB}$
Total charge on both the capacitors is,$
Q=Q_{1}+Q_{2}=V_{AB}(C_{1}+C_{2})$
Or, $Q/V_{AB}=_{}(C_{1}+C_{2})$
So system is equivalent to a single capacitor of capacitance $C=Q/V_{AB}=_{}$ $C_{1}+C_{2}$
So, when capacitors are connected in parallel their resultant capacitance $C$ is the sum of their individual capacitances.
If there are number $n$ of capacitors connected in parallel, then their equivalent capacitance would be,$C=C_{1}+C_{2}+…….+C_{n}$
Series combination:
Following diagram shows two capacitors of capacitance $C_{1}$ and $C_{2}$ connected in series between points A and B
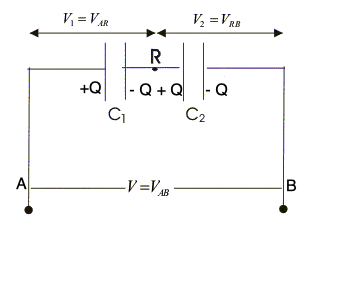
Figure:9.f
Points A and B are maintained at constant potential difference V$_{AB}$.
In series combination of capacitors, all the capacitors would have same charge.
Now potential difference across individual capacitors are given by$V_{AR}=Q/C_{1}$and $V_{RB}=Q/C_{2}$
Sum of $V_{AR}$ and $V_{RB}$ would be equal to applied potential difference $V$.
Therefore,
$V=V_{AR}+V_{RB}$
$V=Q/C_{1}+Q/C_{2}$
$V=Q(1/C_{1}+1/C_{2})$
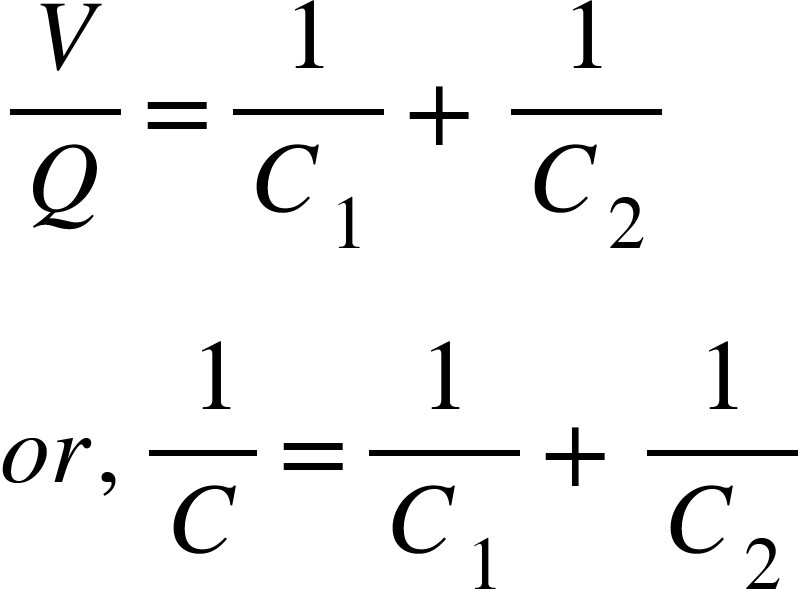
Thus, the resultant capacitance of series combination is equal to the reciprocal of their individual capacitances and $C$ is always less than the smallest individual capacitance.