Concept of force:
A force is a push or pull which changes or tends to change the state of rest or of uniform motion or direction of motion of any object.
The SI unit of force is Newton (N).
The force with which the earth attracts a 1 kg body towards its centre is called kilogram force.
1 kgf = Force in newton / g
The dimensional formula of force is $\lbrack MLT^{-2}\rbrack .$
Fundamental forces:
Gravitational force: The force of interaction that exists between any two objects of masses $m_{1}$and $m_{2}$ is called gravitational force.
Mathematically,
![]()
Where ${r}$ is the position vector and = Universal gravitational constant.
Electromagnetic force: Force exerted by one particle on the other because of the electric charge on the particles is called electromagnetic force.
Nuclear force: It is the strongest force and keeps nucleons together inside the nucleus.
Weak force: It acts between any two elementary particles. A neutron can change into a proton emitting an electron and an antineutrino.
Contact Force:
Contact force is a force that is applied by objects in contact with each other.
Frictional Force: Friction is a force exerted by a surface against the motion of a body across its surface.
Applied Force: Force which is applied to an object by another object. For example, a person pushing a table is an example of applied force.
Normal Force: The normal force is the support force exerted upon an object that is in contact with another fixed or stable object. For example, if a brick is resting on a surface, then the surface is exerting an upward force on the brick in order to support the weight of the brick.
Newton’s first law of motion:
Newton’s First law of motion says that a body would continue to be in a state of rest or of uniform motion in a straight line unless acted upon by a net external force.
The net external force acting on the body is the vector sum of all the external forces acting on the body.
For a body at rest or uniform velocity, the acceleration is zero, hence force is also zero.This implies that for
When the net force is zero, the body is said to be in equilibrium. Thus for a body to be in equilibrium, it can be expressed as $\sum{}F=0$ or $\sum{}F_{x}=0$ and $\sum{}F_{y}=0$
Inertia:
The inherent property of objects to remain at rest unless acted upon by a force is called inertia of rest and the inherent property of objects to remain in a state of uniform motion is called inertia of motion.
Therefore, the property of an object to remain in a state of rest or uniform motion unless acted upon by a force is called inertia.
Mass is the measure of inertia. For example if an equal amount of force is applied on two objects of masses $m_{1}$and $m_{2}$, provided $m_{1}$$>$ $m_{2}$, then acceleration of both the objects would be different. Acceleration of object having larger mass would be lesser then the acceleration of object having smaller mass.
Thus larger the mass of the body ,smaller would be the acceleration and larger would be the inertia.
Newton’s second law of motion:
Newton’s second law of motion gives a relation between force and acceleration. Before we establish this relation, let us define momentum.
Momentum: Momentum ($p) $of a body is the product of its mass $m$ and velocity $v$.
$p=m v$
It becomes obvious that an object has a greater momentum if both its mass and its velocity are large. Both variables are of equal importance in determining the momentum of an object. Consider a truck and a motorbike travelling at the same speed. The greater mass of the truck gives a greater momentum than compared to that of the motorbike.
Second law of motion:
The rate of change of momentum of a body is directly proportional to the applied force and takes place in the direction in which the force acts.
Let ${v}$ be the instantaneous velocity of an object of mass $m$.
Momentum of the object is given by,
![]()
According to the second law,
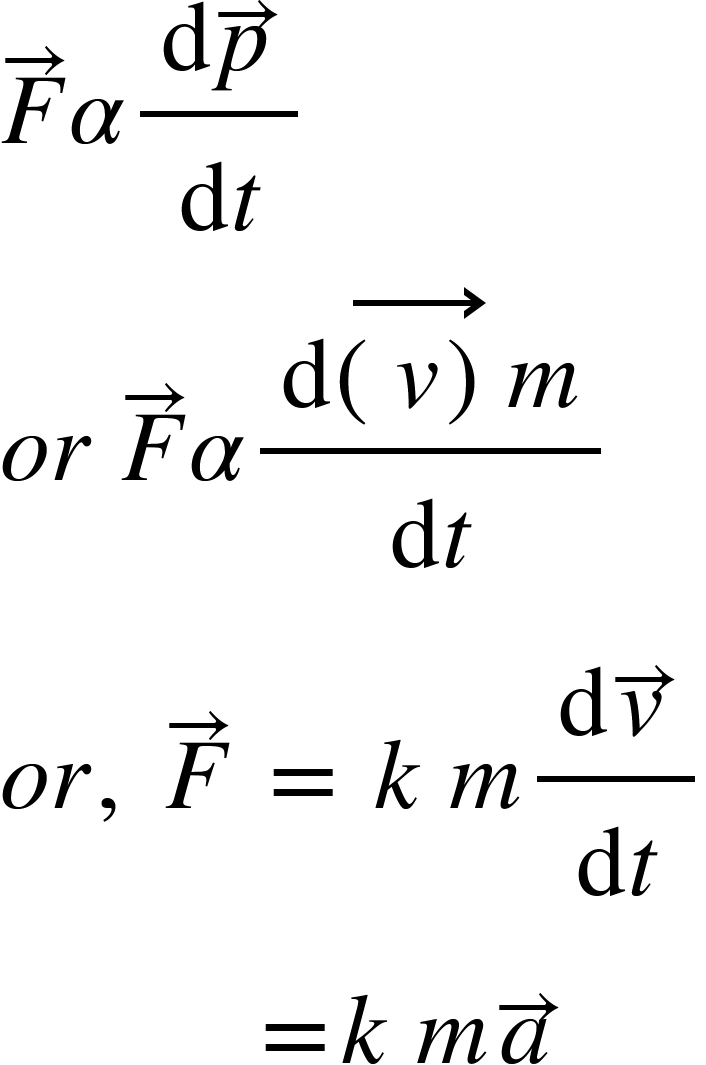
Where ${a}$ is the acceleration of the body and $k$ is the constant of proportionality.
The units of force are selected in such a way that ‘$k$’ becomes one.
Thus, if a unit force is chosen, which produces a unit acceleration in a
unit mass,
i.e., $F=1, m=1 $and $a=1$.
Then, $k=1$.
Therefore, Newton’s second law can be written as,
![]()
The components of force along the X, Y and Z directions are,
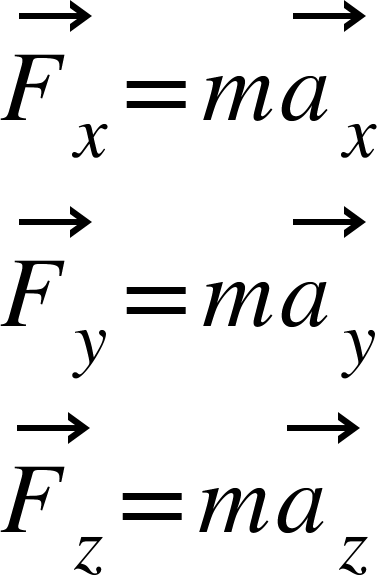
Third law of motion:
To every action there is an equal and opposite reaction.
For example, While walking a person’s foot pushes (which is action) the ground in the backward direction. The ground pushes (which is reaction) the person in the forward direction with an equal force. The component of reaction in the horizontal direction makes the person move forward.
Free Body Diagram (FBD):
A free body diagram consists of diagrammatic representations of a single body or a subsystem of bodies isolated from surroundings showing all the forces acting on it.
Let us draw FBD for given systems.
FBD 1 : There are two forces acting on a block at rest on a table.
- The weight W exerted by the earth on the block.
- The normal force N exerted by the table on the block.
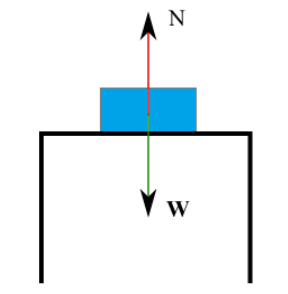
Figure:11.a
FBD 2 : There are two forces acting on the suspended block at rest.
- The weight W exerted by the earth on the block.
- The tension force T exerted by the string on the block.
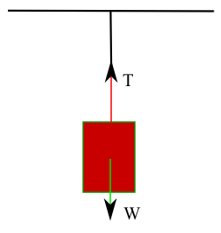
Figure:11.b
FBD 3: The block is pulled by the force $F_{a}$ and therefore a force of friction acts on the block. So there are four forces acting on the block.
- The weight W exerted by the earth on the block.
- The normal force N exerted by floor on the block.
- The acting force Fa to pull the block.
- The force of friction Ff exerted by floor on the block in the direction opposite the motion due to Fa.
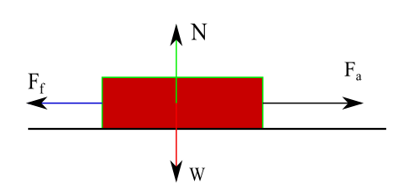
Figure:11.c