A restorative force is always associated with the simple harmonic motion that is acting in the opposite direction of velocity. During the oscillations, the restorative force is changing and is always dependent on the position of the object. In the pendulum, the restorative force is the component of gravity along with the path of the motion. For the pendulums with the increase in the length, the periods also get larger.

Motion of Simple Pendulum
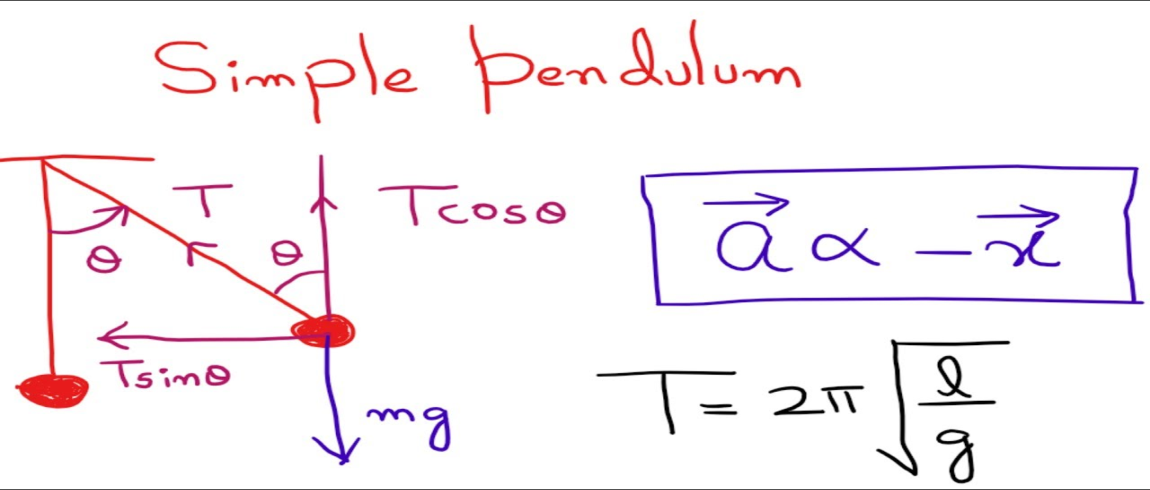
The simple pendulum is the idealized body that consists of the particle which is suspended by the light and inextensible cord. When this object is pulled to the one side of its equilibrium and then it is released the pendulum undergoes the swing in the vertical plane, as it is influenced by gravity. This kind of motion is oscillatory and periodic. The simple pendulums are also used as examples of the simple harmonic motion, as the motion of the simple pendulums is also periodic. They are also fit according to the criteria that the velocity of the bob is maximum when it passes through the equilibrium state and its acceleration is minimum at both endpoints.
Assumptions for Derivation of Expression of Period of Simple Pendulum
For the derivation of the time of the simple pendulum, let consider that a point mass is suspended from the end of the inextensible and light string and its upper end is fixed with the rigid support. With the movement, there is the displacement of the mass from its mean position. But few assumptions are followed for the derivation of the expression of the time of the simple pendulum. These assumptions are as follows.
- There is always negligible friction from the system and air.
- The arm of the pendulum is massless and it does not compress or bend.
- The pendulum swings in the perfect plane.
- Gravity is always there but it remains constant.
For the derivation, the equation of the motion is used.
T – mg cosθ = mv2L
The resultant torque tends to bring the mass to the equilibrium position.
τ = mgL × sinθ = mgsinθ × L = I × α
For the smaller angles of the oscillations the sin ≈ θ
Due to this reason it can be stated that the Iα = -mgLθ
α = -(mgLθ)/I
– ω02 θ = -(mgLθ)/I
ω02 = (mgL)/I
ω20 = √(mgL/I)
Here by using the I = ML2, where I is used to denote the moment of inertia of the bob.
By using the above equation, the result obtained will be ω0 = √(g/L)
Therefore, the time of the simple pendulum can be given as
T = 2π/ω0 = 2π × √(L/g)